Calibration and Analysis of Flowmeters
by legosarekool in Teachers > University+
112 Views, 0 Favorites, 0 Comments
Calibration and Analysis of Flowmeters

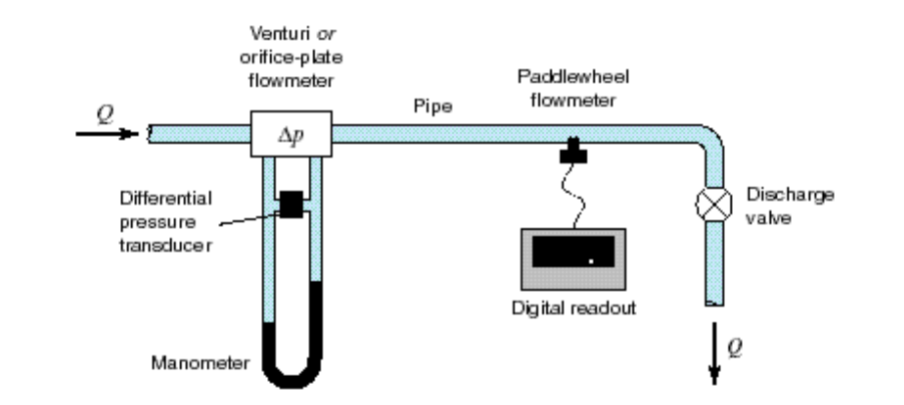
Understanding how a flowmeter works is essential to knowing how to calibrate a flowmeter. A flowmeter measures the flow rate and expresses it as a function of voltage V. Different flowmeters can measure different properties of a flow in order to determine the flow rate. However, if the flowmeter is not calibrated, it may give a reading that is inaccurate. Inaccuracies may also arise as some flowmeters have a range of accuracy and may not be accurate at certain extrema.
Supplies
Supplies:
1 A flow source / fluid flow system
2 Venturi or Orfice-plate flowmeter
3 Paddlewheel flowmeter
Transducer Calibration
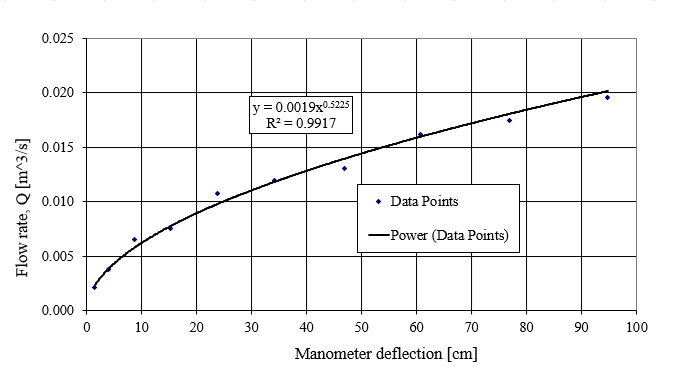
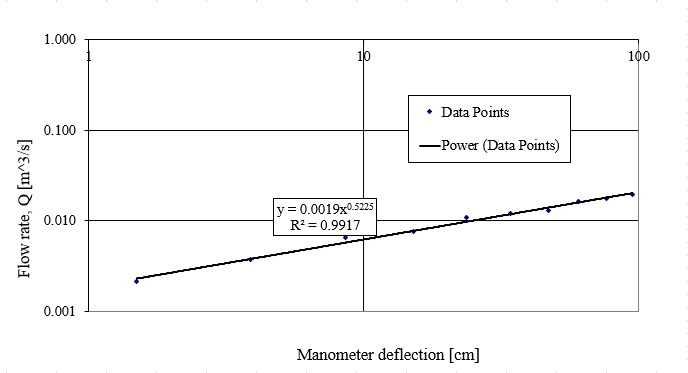
To determine the experiment's validity, it is important to calibrate the traducer voltage to the differential pressure measured by the flowmeter. For this specific transducer, the flow rate was adjusted such that the transducer voltage was approximately 8V. The differential manometer heights were then recorded as the traducer voltage was decreased according to a known scale. (8V (max), 0.9^2*(max), 0.8^2*(max) ... etc). The results for calibration are shown above in the linear and exponential graphs of flow rate vs differential manometer height. Note that for both graphs there is a trendline that fits the data suggesting that a power-law relationship exists between the flow rate and manometer deflection.
Data Gathering

Once the data from Step 1 is gathered. The voltage measured in subsequent flow rates can be used to determine an unknown flow rate using the line of best fit. A paddlewheel flowmeter was put in series farther down the system to also get measurements in voltage (V).
Discharge Coefficient & Reynolds Number
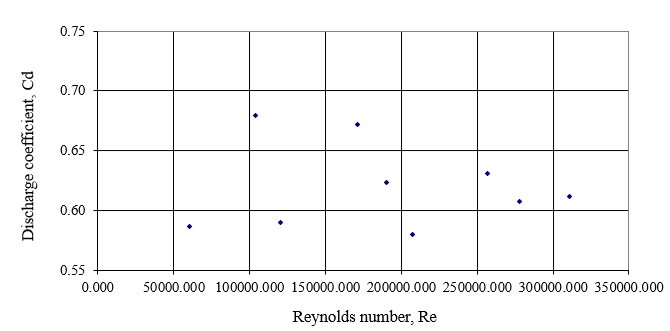
It should be noted that when plotting the discharge coefficient, against the Reynolds number there exists a relationship where the discharge coefficient merges to an approximately equal value when the Reynold number is large. The opposite effect happens when Reynolds number is smaller, the discharge coefficient differentiates.
Further Analysis

The discharge coefficient differed as the Reynolds number changed. As Reynolds number increased, the discharge coefficient converged to approximately 0.62. A more realistic value could be obtained by selecting a material and fluid that would have less loss to friction and turbulent flow increasing the discharge coefficient.
The paddlewheel flowmeter was seen to be fairly consistent across the range of flow tests. It should be noted that due to the physical construction of the flowmeter, it is not accurate when the flow in the system takes up a very small percentage of the pipe area. This is because the paddlewheel is not fully submerged by the flow.