Force Between Magnets

Courses in electromagnetism always start with Coulomb’s law, stating that the force between two electrical point-charges is proportional to the product of their respective charges and inversely proportional to the distance between them.
But what about magnets? We’ve all felt with our own hands the force between magnets, do they also follow the ‘inverse square law? Let’s find out!
Materials required:
- A precision scale, minimum accuracy 0.01 grams
- Two magnets
- An empty plastic candy box
- A wooden skewer
- A pencil
- Cellotape
Setup


We will use the precision scale to measure forces between magnets, but the scale probably contains iron, so we use an empty plastic candy box as a distantiator.
Using a ruler or 5mm-square paper, mark lines on the wooden skewer every 5mm, thicker line every 10mm and a very thick line every 5cm. Stick the skewer into the plastic box. Leave a thick line at a height of half the thickness of the magnet: we’ll measure the force between the magnets as a function of the distance of their centers!
Tape one of the magnets to the top of the box. Tape the other magnet to the end of a pencil.
Measure



Place the candy box with skewer and magnet on the scale. Push ‘TARE’ or ‘TARA’ or similar to reset the scale to zero.
Carefully approach the pencil with the other magnet from the top, such that they are well aligned, but without any direct contact. At a certain distance, you will see that the scale starts to show a non-zero number. In case of a positive number, the magnets repel, in case of a negative number, the magnets attract. Either is fine, you should redo the measurement with the opposite configuration.
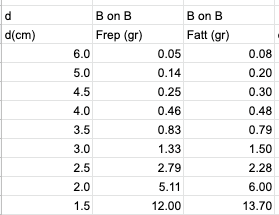
Decide the maximum distance for which you find a sensible reading, in my case 6 cm. Note down the force on a piece of paper or directly in a spreadsheet. Bring the magnet closer in steps of 0,5 or 1,0 cm and note down the force. You can repeat this measurement for different magnet combinations.
Make a Graph
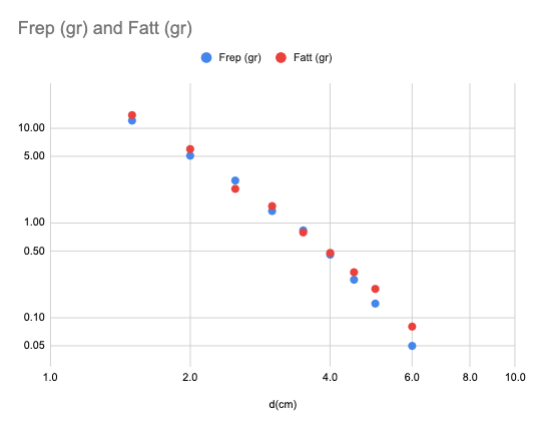
In Google sheets or Excell, you can make a plot of force vs distance, but it will just show that the force decreases very quickly for increasing distance. How do we verify if the measured curve follows the inverse-square law? The best is to use double-logarithmic scales. A power-law dependence will show up as a straight line on a plot where both the horizontal and the vertical axes are logarithmic. In Google sheets, this can be done by choosing ‘scatter chart’ and go to ‘customize’ then ‘horizontal axis’ or ‘vertical axis’ and tick the option “logarithmic scale” for both.
Analyze
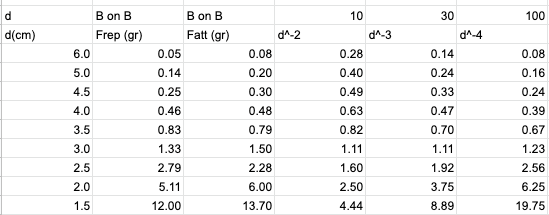
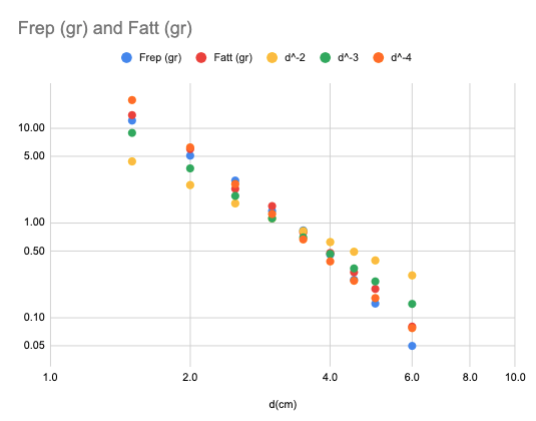
In principle you’d like to make a linear fit to the data points, but I don’t know how that works in Google sheets, so the next best thing is to compare the data to theory, and adjust the theory parameters by hand until they match the data. So we add a column in the sheet next to the data, and fill it with numbers that follow the inverse square law. Whatever we try, the data won’t fit, the magnetic force decreases much more strongly than with the inverse square of the distance. We add a column with d^-3. It is much better but not great. Another column with a d^-4 dependence describes the data very well.
Theory
So we find that the force between magnets decreases with the fourth power of the distance. Was this to be expected? There are not many electromagnetism courses and books which handle this topic, but they all provide the basic ingredients to understand that the force between magnets follow a d^-4 law. Here are two ways to understand:
Magnets are dipoles, and even though they do not contain a separated positive and negative magnetic charge like an electric dipole does, at large distance, the fields behave as if they do. So we can see the force of two magnets as the force between two electric dipoles. The dipole field decreases as d^-3 and if we put another magnet in such a field, with again two lightly separated charges, they will each fell a force that differ by (d-Delta)^-3-(d+Delta)^-3, which can be shown to behave as d^-4.
Similarly, but more precisely, we can treat the magnets as true magnetic dipoles, with a dipole moment M. The field on the symmetry axis at large distances is B=mu0 M / (2*pi*d^3). The force of another dipole in this field follows from the space derivative of its potential energy: F=-dU/dz=mu0 M1M2 *3/(2pi) d^-4.
Conclusion and Bonus
So we conclude that we have demonstrated that the force between magnets diminishes with the 4th power of the distance, as expected by the complete theory, and definitively different from Coulombs law for electric charges. The measurement is straightforward and can be repeated and varied with simple means and little effort.
As a bonus, this method can be used to quantify the strength of a magnet in terms of its magnetic moment, as long as we have two identical magnets. The equation from the previous step can be inverted, and for the case of identical magnets becomes M=sqrt[2piFd^4/(3mu0)].
Converting the numbers from this measurement to SI units and filling them in, we find:
Fd^4 = 100 gr g cm-4 = 10^-8 Nm^4
M=sqrt[(2*pi*10^-8)/(3*4*pi*10^-7)]=0,16Am^2
The magnet has a thickness of 2,9 mm and a diameter of 17,3mm, and therefore a volume of 0,68x10-6 m^3.
The magnetisation thus results 2,3x10^5A/m